Sample Course Content Maths - Integers
Here is the Sample Course Content – Maths : Integers. Please note that this is only a sample content and not the full fledged one.
When you play hide and seek, while all children run to hide, one player is expected to count 1,2,3……..up to 20. Did you notice, we do not count in decimals or part of a number like two and a half?
All the numbers which are whole in nature (not part or fraction) , either positive or negative are called Integers. E.g. 1,2,3…. Or -1, -2,-3…..
So, is 0 (zero) an integer? Find out.
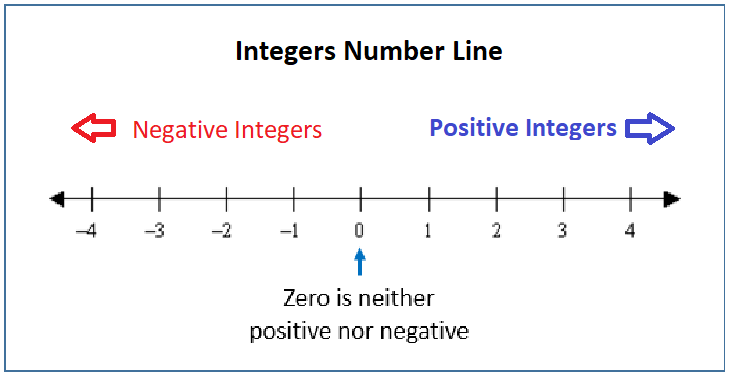
Integers are shown in ascending/descending order as:
As you will notice in the diagram above, value of an integer value goes
· up while moving from left to right
· down while moving from right to left
even for negative integers, the same hold true. So, -7 is smaller in value than -6
Remember, every positive integer has a negative counter part and these are called additive inverse of each other. E.g. -3 is additive inverse of 3
We can do multiple mathematical operations on integers. However, for now, we will limit ourselves to basic operations.
Rules of multiplication
Rule # 1: Product of two integers of opposite sign is equal to additive inverse of product of their absolute values
e.g.
a. -14 X 3 = – (14 X 3) = -42
b. -6 X 10 = – (6 X 10) = -60
c. 4 X -3 = – (4 X 3) = -12
Now, do the exercise related to Rule # 1 before you proceed
Rule # 2: Product of two integers of same sign is equal to the product of their absolute values. E.g.
a. 2 X 4 = 8 ; 3 X 6 = 18
b. -2 X -4 = 2 X 4 = 8
c. -3 X – 6 = 3 X 6 = 18
Now, do the exercise related to Rule # 2 before you proceed to the next section
1. Closure rule of addition and multiplication
The rule states that: “Sum or product of two integers will always be an integer”
Multiplication Addition
a. 4 X 3 = 12
b. -7 X 6 = -42
c. -8 X -9 = 72
d. 4 + 3 = 7
e. -7 + 6 = -1
f. -8 + -9 = -17
All the numbers mentioned above including multiplicands and products are integers
2. Commutative rule of addition and multiplication
Addition and multiplication of two integers is commutative in nature”
a. 3 X 4 = 4 X 3
b. 7 X – 6 = – 7 X 6 = -(7 X 6)
Division is repetitive subtraction. It is inverse of multiplication.
e.g. 20 / 5 = 4; -20/5 = -4
Dividend: The integer, that is to be divided is called dividend. For example in the examples above, 20 is dividend.
Divisor: The integer, which divides is called divisor. In the example above 5 is divisor
Quotient: The result of division is called quotient. In the example above, 4 is quotient
Rule 1: if either dividend or divisor is negative, quotient will be negative.
e.g. -20/5 = -4 and also 20/-5 = -4
Rule 2: If both dividend and divisor have same sign (+ or -), quotient will be positive
Exercise:
a. 8/4
b. -10/2
c. 9/3
d. -8/-2
e. 21/7
f. -81/9
g. -63/7
h. 72/24
i. -90/10
-54/6
Operator Precedence
In case of multiple operations, we need to set order of operation. The order of operation is DMAS.
· D = Division
· M = Multiplication
· A = Addition
· S = Subtraction
So, in case of multiple operations, we will first divide, then multiply followed by addition and subtraction
e.g. if we get a sum as: 8+2×3-9/3
our order of operation will be:
8+2×3-9/3 = 8+2×3-3 = 8+6-3 = 14-3 = 11
Using Bracket to decide precedence
If in a sum, we want to prioritize some operation, out of generic precedence we use brackets. e.g. in the sum above, if we use bracket to prioritize subtraction:
8+2x(3-9)/3 = 8+2x(-6)/3 = 8+2x(-2) = 8+(-4)= 8-4 = 4
See the difference a bracket can bring into mathematical operation
There are three types of brackets
( ) è Parenthesis or common bracket – this is used most commonly for operations
{ } è Braces or curved bracket
[ ] è Square brackets or Box bracket
These brackets are used as:
28 – [7 + {8 – (1+2)}]
= 28 –[7 + {8 – 3}]
= 28 – [7+5]
= 28 – 12
= 16
In this lesson, we learnt
1. All numbers, which are not decimal or fraction are called Integers
2. There are positive and negative integers
3. Integers can be added, subtracted, multiplied or divided by/with another integer
4. Operations on integration follow precedence of DMAS
5. Brackets can be used to re-prioritize operation

